RETURN to Periodic Table
Below: Electron Shell, Bonding & Ion Formation, Magnetic Properties, Electric Properties
Copper is the 29th element on the periodic table. It has 29 protons and 34 neutrons for a mass of 63.5 amu, and 29 electrons.
Electron Shell 
DISCLAIMER: The following reflects the sub-quantum mechanics approach to electron interactions and hybridization. Some details may therefore differ somewhat from traditional quantum chemistry:
Copper is the ninth element with electrons in the d–orbital. Building upon the pd-hybridization [ref] we introduced in regard to the previous d-block transition metals, it is proposed that copper features p3d5-hybridization. This enables a symmetrical, eight-directional cubic geometry with 8 di-electrons (see image below).
This pd-hybridization does not need to involve the 3s-orbital electrons, so they can retain their preferred spherical di-electron state. However, it is achieved in the same way as we saw with chromium, where one electron from the 4s-orbital joins the pd-hybridization in order to achieve 8-directional symmetry and lowest energy. (In the views below, this cube configuration is tilted to reveal that it is also a double-tetrahedral arrangement — two tetrahedra aligned antiparallel.)
The 3rd shell cubic di-electrons will arrange their double tetrahedra in such a way that minimizes repulsion with the 2nd shell di-electrons beneath (as depicted in the images below).

 CLICK HERE to interact with this object
CLICK HERE to interact with this objectNOTE: The small spheres in the image above simply indicate the directions of maximum electron density. The 3rd shell hybrid orbitals themselves will form a cubic arrangement that divides the (cuboctahedral) shell into eight equal volumes. Each shell segment will be filled with electron density. It will be highest at the center of the face of each orbital (as in the traditional hybrid orbital lobe shapes) and will decrease toward the nodal regions between orbitals — as wave structures usually do — where electron density will be lowest (though not necessarily zero).
NOTE ALSO: Even though it is often useful to talk about these orbitals as separate, they are all — the entire atom is — part of a single, coherent, harmonic, resonant, phase-locked, spherically-symmetrical quantum wave state, and it is all electromagnetic at the root-energy level. Orbitals and their ‘boundaries’ can be seen as nothing more than nodes and antinodes in this harmonic wave structure.
As in the case of the previous (transition metal) elements, copper’s 3rd shell p3d5-hybrid orbitals exist within the added sheath of the 3s2-orbital di-electron, since it is not needed for hybridization. It is proposed that they are superimposed within it, as a harmonic frequency coincides with its fundamental frequency in a resonance. It is suggested that the 3s2 di-electron gives additional stability to the electron configuration within it. The other elements in the d-block will also experience this 3s-orbital stabilization phenomenon, since they achieve at least 4-directional symmetry with only their p– and d-orbitals.
Observational evidence may bear out the proposed cubic structure of copper’s 3rd shell. The following images of copper’s Fermi surface were obtained by Weber (et.al.) using a process known as 2D-ACAR. [Ref]

Bonding & Ion Formation 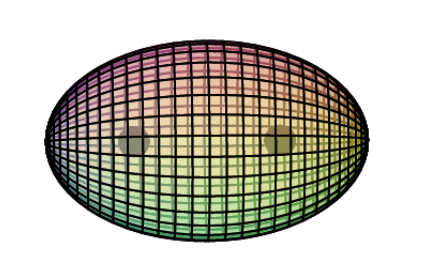
As mentioned above, a 4s1 electron shell completes the atom. This is because one of the valence electrons drops down into the 3rd shell hybridization in order to allow it to achieve 8-directional symmetry. (This occurs in a few other cases, for example chromium (Cr) and silver (Ag).)
When forming a metallic crystal, the 4s1 electron delocalizes to form the metallic bond, and when forming a 1+ ion, it is removed. In both cases, the core electron geometry remains the same.
A second ionization, however, would shift its geometry, perhaps by ionizing one of the hybrid di-electrons. This would retain 8-directional symmetry, but with one of the hybrid orbitals now containing an unpaired electron. It would therefore be expected that the Cu2+ ion would be strongly paramagnetic (see below) — due to its single and highly constricted unpaired electron orbital — and the Cu+ ion would be diamagnetic. They are. By way of example, CuCl has χm = –40 while CuCl2 has χm = +1,080.
Magnetic Properties 
Copper is diamagnetic.
DIAMAGNETISM:
Diamagnetism occurs when a substance is repelled from a magnetic field because it contains di-electrons. These paired electrons are in a state of field cancellation with one another. The presence of an external magnetic field will disrupt the coherence of their di-electron states, raising energy. This causes the atom to repel away from the field in search of a lower energy state. Diamagnetic substances have negative magnetic susceptibility (χm) values.
Copper’s 3rd shell is full, with a symmetrical arrangement of 8 field-repelling di-electrons. While it does have one unpaired 4s1 electron in its valence shell, it is presumed that the diamagnetism of these di-electrons overpowers any paramagnetism due to the 4s1 electron, giving copper a magnetic susceptibility of χm = –5.46.
Zinc, with a full 4s2 orbital, has an even lower magnetic susceptibility of χm = -9.15. This may imply that the presence of copper’s 4s1 electron may be disrupting the diamagnetism of its inner symmetrical arrangement of 8 field-repelling di-electrons., decreasing their diamagnetism slightly with respect to an external magnetic field.
MAGNETIC STRENGTH ANALYSIS:
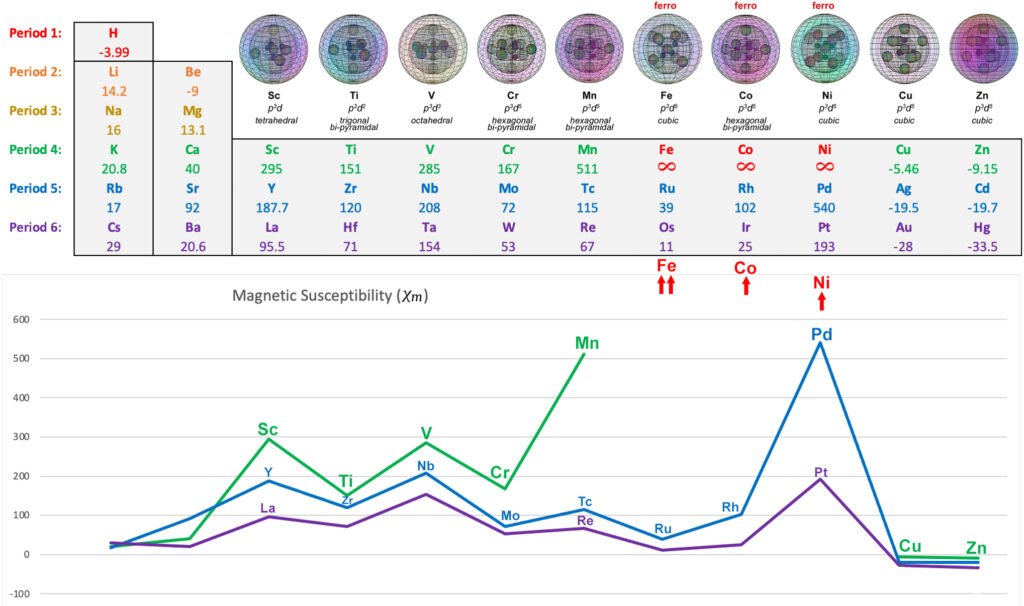
The following diagram shows the relative paramagnetic and diamagnetic strengths of the transition metals, along with their proposed hybrid orbital geometries. (See Magnetism for more detail.)
The following video shows some of the remarkable effects of copper’s resisting the approach of a strong magnetic field. Watch as a strong magnet is passed quickly over or quickly towards a slab of copper.
Electrical Properties 
Copper is the second most electrically conductive metal after silver (Ag), and gold (Au) is third.
Not only are the three in the same group within the d-block, but they also have in common a valence s-orbital that has donated an electron inwards in order to perfect the symmetry of an inner cubic di-electron shell.
We might speculate that such an ‘activated,’ electron-deficient valence conduction band may actually promote the passage of electrical potential. It may simply offer less resistance to it (by having more unoccupied energy states in the band structure that are available to transmit electrons).
However, chromium also has a deficient 4s orbital, but its electrical conductivity is about 10 times smaller than those of the precious metals. We might further speculate that, what separates the three precious-metal good conductors from chromium is the nature of their core electron resonances. In the three precious metal elements, the inner orbitals are all completely filled with di-electrons, which are diamagnetic. We might speculate then that, upon this ‘non-interfering’ foundation, the single-valence-electron conduction band may support the transmission of electrical potential through the metallic crystal quite effectively. In the case of chromium, however, there are five unpaired electrons on the surface of every atomic core in the crystal. Their magnetic field and spin interactions might interfere with the electrons in the conduction band in such a way that diminishes conductivity, due to resistance. It is further conceivable that, given the ratio of electrons involved, it may decrease chromium’s conductivity to merely one tenth of the conductivity of copper and silver.
Credence for this conjecture might come from the fact that, even though chromium has a much lower electrical conductivity than copper, it nevertheless has a much higher conductivity than its immediate neighbors in the 3d-block — vanadium (V) and manganese (Mn) — which each have full valence 4s2-orbitals. (Though this fact is not conclusive on its own.)
PARAMAGNETIC 3d METALS: Scandium, Titanium, Vanadium, Chromium (also antiferromagnetic), Manganese
FERROMAGNETIC 3d METALS: Iron, Cobalt, Nickel
OTHER DIAMAGNETIC 3d METALS: Copper, Zinc
RETURN to the Periodic Table